Tangent-Asymptote triangle of a Hyperbola, Part 1

While exploring a different area of math, I came across the fact that a tangential segment of a hyperbola between the asymptotes is bisected at the tangent point.

Several attempts and internet searches later, I realised that this was already known to Apollonius of Perga. Reading the Conics, it became clear Apollonius proves this through a series of propositions.

While it was a great read, I was a little disappointed at the length of the proof and wanted to find a shorter one. The content of this post consequently is the proof that I found in this regard.

Consider the hyperbola $y^2-x^2=1$. Take any point $B$ on the upper branch and draw a tangent at that point. Draw a line parallel to the tangent such that it cuts upper branch of the hyperbola at two points as shown in the following figure. We show that the midpoint of $DE$ coincides with that of $FG$.

Figure 1

The idea of the proof is to use the third dimension as well. We know that the hyperbola is the intersection curve of a cone and a perpendicular plane. 

Figure 2

If we now consider the line through $EF$ as a plane in the above figure cutting the cone, giving an ellipse as shown in the following figure. Note that this ellipse also cuts the hyperbola.

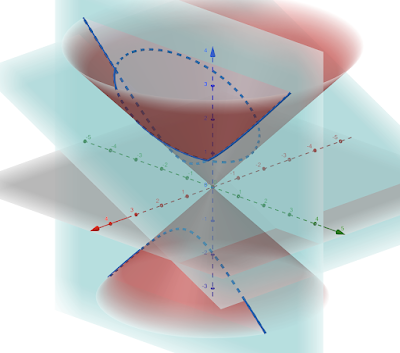Figure 3

Looking from the $x$-axis, this arrangement would look like the following figure.

Figure 4

Looking through the line perpendicular to the plane containing the ellipse, it is clear that the tangent segment caught between the asymptotes is the major of the ellipse and the segment caught between the hyperbola is a chord parallel to the major axis of the ellipse. It is clear then that the midpoints of both the segments will lie on the minor axis.

Figure 5

But looking through the line perpendicular to the plane containing the hyperbola, the minor axis is just a point proving that the midpoint of both the segments coincide.

Moving back to 2D, this property is true for all the line cutting the hyperbola. In the limiting case of a tangent line to the hyperbola, the segment caught between the hyperbola collapses to a point which is its own midpoint and therefore the midpoint of the tangential segment caught between the asymptotes.

This is very nice as we can use it give a geometric proof of $x^{-1}$ which I wasn't able to find even after an extensive internet search.

Note that Apollonius showed something more. He also proved for a given tangent, the midpoints of all the chords parallel to the tangent lie on a straight line passing through the center of the hyperbola and too for all the conics. Truly spectacular!

Until then
Yours Aye
Me